Let Joy Be Your Guide!
What do you notice? What do you wonder?
This is a guide for parents and caregivers who want to help players discover the underlying mathematical structure behind the MULTI™ board game.
First off, we think it’s important to say, it is perfectly fine to just play MULTI, with no other goal than to have fun!
In fact, if you let joy be your guide, you will undoubtedly be doing mathematics, plain and simple.
That said, we wanted to dive a bit into some topics that are likely to arise and questions that can help draw out the mathematics naturally embedded in the game.
The set of questions below are designed to be easy to begin to explore, with enough depth to challenge even the most veteran mathemagicians amongst us.
The structure of multiples and factors are front and center in this game! However, depending on the player’s familiarity with numbers, the structure may be hidden at first, like a map hidden in plain sight.
If you’d like to dive deeper into mathematics you can start by focusing on any of the following questions, based on interest and familiarity with the concepts.
These questions are meant to be conversation starters, and the answers I include are by no means comprehensive.
Spoiler Alert: We have included some possible answers to each question. If you want to explore a question without any clues, just ignore the italics text under the questions.
Here’s a list of the key topics that could come up for players in different age ranges.
Key Topics
EARLY ELEMENTARY (K-2)
Arrays, rectangles, area, square units, etc.
Square Numbers
Recognizing patterns and structure
Symmetry
Strategy in general, openings and endgame situations
UPPER ELEMENTARY (3-5)
Arrays, rectangles, area, square units, etc.
Square Numbers
Recognizing patterns and structure
Symmetry
Strategy in general, openings and endgame situations
Multiples, common multiples
Factors, common factors
MIDDLE SCHOOL AND BEYOND
Arrays, rectangles, area, square units, etc.
Recognizing patterns and structure
Symmetry
Strategy in general, openings and endgame situations
Multiples, common multiples
Factors, common factors
Combinatorics
Game Theory
Computer Science, coding, machine learning, etc.
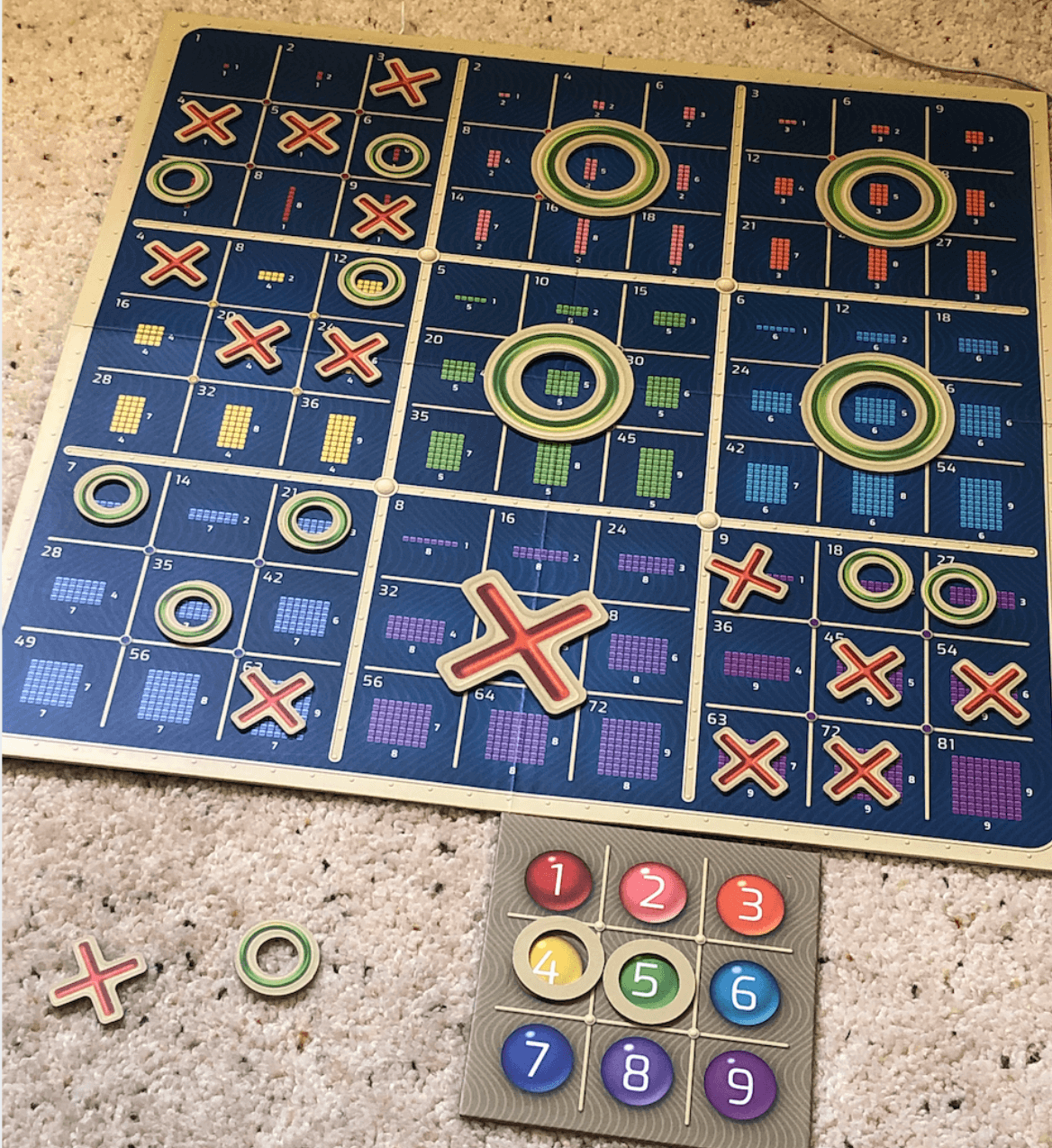
Patterns on the MULTI Board
Which numbers come up the most? Which numbers come up the least? Why?
There are single, double, triple, and quadruple plays. For example 1, 25 and 81 only come up once, and 12, 18, and 24 come up four times! In the picture on the right, you can see how 18 is a quadruple play. The numbers that come up four times have two different pairs of factors(on the factor board), for example 18 is 2x9, 9x2, 3x6, and 6x3, showing up in the two’s, three’s, six’s, and nine’s mini boards.
Can you find all the quadruple plays?
Try placing black Xs on all the quadruple plays, red Xs on the triple plays, white Os on the double plays, and green Os on the single plays.
What are some of the best quadruple plays? Why?
Answers will vary depending on the situation, playing style, and preference.
Multiple Symmetry
The 4th multiple in each mini tic tac toe board mirrors the location of multiples of 4 on the big board.
Where are the multiples of 4?
The multiples of 4 are in the middle row on the far left of the entire MULTI board, but also in the same spot on each of the mini tic tac toe boards. This pattern is highlighted in the picture to the right. There are a few extra multiples of 4, can you find them?
Why does this pattern exist?
These patterns exist because each multiple of 4 is the 4th multiple of another number, and therefore would be on the left of the middle row.
What multiples are in the centerboard? Where else are those numbers?
Multiples of 5, they are in the center of each mini board as well.
Where are the square numbers? How many times do each of them show up?
The square numbers are found at 1x1, 2x2, 3x3, 4x4, 5x5, which gives you the 1st number in the One’s Board, and the 2nd number in the Two’s board, 3rd number in the Three’s Board, etc. The numbers 1, 25, 49, 64, and 81 are single plays, while 4, 9, 16, and 36 are triple plays.
What Moves are possible in this turn?
Sometimes players may get stuck, and aren’t sure what to move. Its useful to narrow down what to consider, by thinking about what moves are possible. For example if the tokens are on 4 and 7, you only need to consider multiples of 4 and multiples of 7, that have not yet been claimed. At the beginning of the game, that may be 7 x(1,2,3,5,6,7,8, and 9) and 4x (1,2,3,4,5,6,8,9), and at the end of the game there may only be a few options.
Strategy Talk
What are the best opening moves? What should you consider?
Answers will vary, but arguments can be made for quadruple plays on the basis of quantity, and an argument can be made for certain locations being more quality moves to start.
How can playing on a square number guarantee you can play on the same MINI Tic Tac Toe board on your next turn? Does it always work?
The Power of Playing on Square Numbers
If you have both tokens on the same number on the Factor Board, then your opponent can only move one off during their turn, leaving you with another possible move with that factor. However, playing square numbers doesn’t always work this way, for example if you play on 36, but you use 9x4 to get it.
How can you prevent a player from getting a square they need for tic tac toe?
There are two main ways to block a player from getting a square they need. The straight forward way is to take the square yourself. However, another way to do it is to avoid placing a token on the factors they need to make the number they need for tic tac toe. For example, say they need to get 15, you would avoid having a token on either 3 or 5, since you can only get 15 by doing 3 x 5.
What are the most important squares/locations?
Answers will vary depending on the situation, playing style, and preference.
ENDGAME
Who has a path to win? What moves are possible?
How far back do you have to go to find a pathway to a different winner?
Take a picture of the game a few steps before the end, and see if you can find an alternate ending to the game.
Who has a path to win?
What moves are possible?
Can either X or O guarantee a win?
Can the game end in a simultaneous win?
We like to call this a true “win-win!”
Challenge Questions
These questions are a bit more involved, and can lead you down some fantastic mathematical rabbit holes involving combinatorics, game theory, and computer science. We’d love to hear from anybody who’s gone down one of these rabbit holes, and learn about what you discovered.